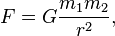Newton's law of universal gravitation

From Wikipedia, the free encyclopedia

Jump to: navigation, search

Physical cosmology

Key topics
Universe · Big Bang Age of the universe Timeline of the Big Bang Ultimate fate of the universe
Early universe
Inflation · Nucleosynthesis GWB · Neutrino Background Cosmic microwave background
Expanding universe
Redshift · Hubble's law Metric expansion of space Friedmann equations FLRW metric
Structure formation
Shape of the universe Structure formation Galaxy formation Large-scale structure
Components
Lambda-CDM model Dark energy · Dark matter
History
Timeline of cosmology...
Cosmology experiments
Observational cosmology 2dF · SDSS CoBE · BOOMERanG · WMAP
Scientists
Einstein · Hawking . Friedman · Lemaître · Hubble · Penzias · Wilson · Gamow · Dicke · Zel'dovich · Mather · Sagan · Smoot · others
This box: view • talk • edit

Isaac Newton's theory of universal gravitation (part of classical mechanics) states the following:

Every single point mass attracts every other point mass by a force pointing along the line combining the two. The force is proportional to the product of the two masses and inversely proportional to the square of the distance between the point masses:

$F = G \frac{m_1 m_2}{r^2},$

where:

F is the magnitude of the gravitational force between the two point masses,

G is the gravitational constant,

m₁ is the mass of the first point mass,

m₂ is the mass of the second point mass,

r is the distance between the two point masses.

Assuming SI units, F is measured in newtons (N), m₁ and m₂ in kilograms (kg), r in metres (m), and the constant G is approximately equal to 6.67 × 10⁻¹¹ N m² kg⁻². G was first accurately measured in the Cavendish experiment by the British scientist Henry Cavendish in 1798, it was also the first test of Newton's theory of gravitation between masses in the laboratory. This was 111 years after the publication of "Philosophiae Naturalis Principia Mathematica" and 71 years after Newton's death, so all of Newton's calculations could not use the value of G; instead he could only calculate a force relative to another force.

Newton's law of gravitation resembles Coulomb's law of electrical forces. Newton's law is used to calculate the Gravitational force between two masses; similarly Coulomb's Law is used to calculate the magnitude of electrical force between two charged bodies. Coulomb's Law's equation has the product of two charges in place of the product of the masses which is in Newton's Law of Gravitation. Hence, according to Coulomb's Law, the electrical force is proportional to the product of the charged bodies divided by the distance between them.

1 Acceleration due to gravity
2 Bodies with spatial extent
3 Vector form
4 Gravitational field
5 Problems with Newton's theory
- 5.1 Theoretical concerns
- 5.2 Disagreement with observation
- 5.3 Newton's reservations
- 5.4 Einstein's solution
6 See also
7 Notes

[edit] Acceleration due to gravity

Let a₁ be the acceleration experienced by the first point mass due to the gravitational force exerted on it by the second point mass. Newton's second law states that F = m₁ a₁, meaning that a₁ = F / m₁. Substituting F from the earlier equation gives:

$a_1 = G \frac{m_2}{r^2}$

and similarly for a₂.

Assuming SI units, gravitational acceleration (as acceleration in general) is measured in metres per second squared (m/s² or m s^-2). Non-SI units include galileos, gees (see later), and feet per second squared.

The force attracting a mass to the earth also attracts the earth to the mass, so that their acceleration to each other is given by:

$a_1 + a_2 = G \frac{m_1+m_2}{r^2}$

If m₁ is negligible compared to m₂, small masses would have approximately the same acceleration. However, for appreciably large m₁, the combined acceleration, should be considered.

If r changes proportionally very little during an object's travel – such as an object falling near the surface of the earth – then the acceleration due to gravity appears very nearly constant (see also Earth's gravity). Across a large body, variations in r, and the consequent variation in gravitational strength, can create a significant tidal force. For example, the near and far side of the earth are around 6,350 km different distance from the Moon; although a small difference compared to the 385,000 km average separation, this is enough to cause a slightly different gravitational force by the moon on the earth's oceans on each side compared to that exercised on the earth itself, and hence give rise to the tides.

[edit] Bodies with spatial extent

If the bodies in question have spatial extent (rather than being theoretical point masses), then the gravitational force between them is calculated by summing the contributions of the notional point masses which constitute the bodies. In the limit, as the component point masses become "infinitely small", this entails integrating the force (in vector form, see below) over the extents of the two bodies.

In this way it can be shown that an object with a spherically-symmetric distribution of mass exerts the same gravitational attraction on external bodies as if all the object's mass were concentrated at a point at its centre^[1]. (This is not generally true for non-spherically-symmetrical bodies.)

For points inside a spherically-symmetric distribution of matter, Newton's Shell theorem can be used to find the gravitational force. The theorem tells us how different parts of the mass distribution affect the gravitational force measured at a point located a distance r₀ from the center of the mass distribution:

The mass located at a radius r < r₀ causes the same force at r₀ as if all of the mass enclosed within a sphere of radius r₀ were concentrated at the center of the mass distribution (as noted above).
The mass located at a radius r > r₀ exerts no net gravitational force at r₀. I.e., the individual forces exerted by the elements of the sphere on the point at r₀ cancel each other out.

As a consequence, for example, within a shell of uniform thickness and density there is no net gravitational acceleration.

[edit] Vector form

Gravity on Earth from a macroscopic perspective.

Gravity in a room: the curvature of the Earth is negligible at this scale, and the force lines can be approximated as being parallel and pointing straight down to the center of the Earth

Newton's law of universal gravitation can be written as a vector equation to account for the direction of the gravitational force as well as its magnitude. In this formula, quantities in bold represent vectors.

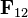$\mathbf{F}_{12} = - G {m_1 m_2 \over {\vert \mathbf{r}_{12} \vert}^2} \, \mathbf{\hat{r}}_{12}$

where

$\mathbf{F}_{12}$ is the force applied on object 2 due to object 1

G

is the gravitational constant

m 1

and

m 2

are respectively the masses of objects 1 and 2

$\vert \mathbf{r}_{12} \vert \ = \vert \mathbf{r}_2 - \mathbf{r}_1 \vert$ is the distance between objects 1 and 2

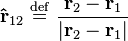$\mathbf{\hat{r}}_{12} \ \stackrel{\mathrm{def}}{=}\ \frac{\mathbf{r}_2 - \mathbf{r}_1}{\vert\mathbf{r}_2 - \mathbf{r}_1\vert}$ is the unit vector from object 1 to 2

It can be seen that the vector form of the equation is the same as the scalar form given earlier, except that F is now a vector quantity, and the right hand side is multiplied by the appropriate unit vector. Also, it can be seen that F₁₂ = − F₂₁.

[edit] Gravitational field

The gravitational field is a vector field that describes the gravitational force which would be applied on an object in any given point in space, per unit mass. It is actually equal to the gravitational acceleration at that point.

It is a generalization of the vector form, which becomes particularly useful if more than 2 objects are involved (such as a rocket between the Earth and the Moon). For 2 objects (e.g. object 2 is a rocket, object 1 the Earth), we simply write 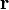$\mathbf r$ instead of $\mathbf r_{12}$ and $m$ instead of $m 2$ and define the gravitational field $\mathbf g(\mathbf r)$ as:

$\mathbf g(\mathbf r) = - G {m_1 \over {{\vert \mathbf{r} \vert}^2}} \, \mathbf{\hat{r}}$

so that we can write:

$\mathbf{F}( \mathbf r) = m \mathbf g(\mathbf r)$

This formulation is dependent on the objects causing the field. The field has units of acceleration; in SI, this is m/s².

Gravitational fields are also conservative; that is, the work done by gravity from one position to another is path-independent. This has the consequence that there exists a gravitational potential field V(r) such that

$\mathbf{g}(\mathbf{r}) = - \mathbf{\nabla} V( \mathbf r)$ .

If m₁ is a point mass or the mass of a sphere with homogeneous mass distribution, the force field g(r) outside the sphere is isotropic, i.e., depends only on the distance r from the center of the sphere. In that case

$V(r) = G \frac{m_1}{r}.$

[edit] Problems with Newton's theory

Newton's description of gravity is sufficiently accurate for many practical purposes and is therefore widely used. Deviations from it are small when the dimensionless quantities φ/c² and (v/c)² are both much less than one, where φ is the gravitational potential, v is the velocity of the objects being studied, and c is the speed of light. ^[2] For example, Newtonian gravity provides an accurate description of the Earth/Sun system, since

$\frac{\Phi}{c^2}=\frac{GM_\mathrm{sun}}{r_\mathrm{orbit}c^2} \sim 10^{-8}, \quad (\frac{v_\mathrm{Earth}}{c})^2=(\frac{2\pi r_\mathrm{orbit}}{(1\ \mathrm{yr})c})^2 \sim 10^{-8}$

where r_orbit is the radius of the Earth's orbit around the Sun.

In situations where either dimensionless parameter is large, then general relativity must be used to describe the system. General relativity reduces to Newtonian gravity in the limit of small potential and low velocities, so Newton's law of gravitation is often said to be the low-gravity limit of general relativity.

[edit] Theoretical concerns

There is no immediate prospect of identifying the mediator of gravity. Attempts by theorists to identify the relationship between the gravitational force and other known fundamental forces are not yet resolved, although considerable headway has been made over the last 50 years (See: Theory of everything and Standard Model). Newton himself felt the inexplicable action at a distance to be unsatisfactory (see "Newton's reservations" below).
Newton's theory requires that gravitational force is transmitted instantaneously. Given classical assumptions of the nature of space and time before the development of general relativity, a propagation delay leads to unstable orbits.

[edit] Disagreement with observation

Newton's theory does not fully explain the precession of the perihelion of the orbit of the planets, especially of planet Mercury^[3]. There is a 43 arcsecond per century discrepancy between the Newtonian prediction, which arises only from the gravitational tugs of the other planets, and the observed precession.
The predicted deflection of light by gravity using Newton's theory is only half the deflection actually observed. General relativity is in closer agreement with the observations.

The observed fact that gravitational and inertial masses are the same for all bodies is unexplained within Newton's system. General relativity takes this as a postulate. See equivalence principle.

[edit] Newton's reservations

While Newton was able to formulate his law of gravity in his monumental work, he was deeply uncomfortable with the notion of "action at a distance" which his equations implied. He never, in his words, "assigned the cause of this power". In all other cases, he used the phenomenon of motion to explain the origin of various forces acting on bodies, but in the case of gravity, he was unable to experimentally identify the motion that produces the force of gravity. Moreover, he refused to even offer a hypothesis as to the cause of this force on grounds that to do so was contrary to sound science.

He lamented that "philosophers have hitherto attempted the search of nature in vain" for the source of the gravitational force, as he was convinced "by many reasons" that there were "causes hitherto unknown" that were fundamental to all the "phenomena of nature". These fundamental phenomena are still under investigation and, though hypotheses abound, the definitive answer is yet to be found. In Newton's 1713 General Scholium in the second edition of Principia:

I have not yet been able to discover the cause of these properties of gravity from phenomena and I feign no hypotheses... It is enough that gravity does really exist and acts according to the laws I have explained, and that it abundantly serves to account for all the motions of celestial bodies. That one body may act upon another at a distance through a vacuum without the mediation of anything else, by and through which their action and force may be conveyed from one another, is to me so great an absurdity that, I believe, no man who has in philosophic matters a competent faculty of thinking could ever fall into it.^[4]

[edit] Einstein's solution

These objections were mooted by Einstein's theory of general relativity, in which gravitation is an attribute of curved spacetime instead of being due to a force propagated between bodies. In Einstein's theory, masses distort spacetime in their vicinity, and other particles move in trajectories determined by the geometry of spacetime. This allowed a description of the motions of light and mass that was consistent with all available observations.

Newton's theory continues to be used as an excellent approximation of the effects of gravity. Relativity is only required when there is a need for extreme accuracy, or when dealing with gravitation for very massive objects.