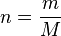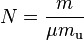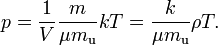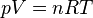Ideal gas law

From Wikipedia, the free encyclopedia

Jump to: navigation, search

Isotherms of an ideal gas

The ideal gas law is the equation of state of a hypothetical ideal gas, first stated by Benoît Paul Émile Clapeyron in 1834.

The state of an amount of gas is determined by its pressure, volume, and temperature according to the equation:

$\ pV = nRT$

where

$\ p$ is the absolute pressure [Pa],

$\ V$ is the volume [m³] of the vessel containing $n\,$ moles of gas,

$\ n$ is the amount of substance of gas [mol],

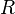$\ R$ is the gas constant [8.314 472 m³·Pa·K⁻¹·mol⁻¹],

$\ T$ is the temperature in kelvin [K].

The ideal gas constant (R) depends on the units used in the formula. The value given above, 8.314472, is for the SI units of pascal cubic meters per mole per kelvin, which is equal to joule per mole per kelvin (J mol^-1 K^-1). Another value for R is 0.082057 L·atm·mol⁻¹·K⁻¹)

"R" has a different value for each different unit of pressure used. The values are... R = 8.314472 (pascals/kPa) R = .0821 (atms) R = 62.4 (torr/mmHg) R = 1.2 (psi)

The ideal gas law is the most accurate for monoatomic gases at high temperatures and low pressures. This follows because the law neglects the size of the gas molecules and the intermolecular attractions. Obviously the neglect of molecular size becomes less important for larger volumes, i.e., for lower pressures. The relative importance of intermolecular attractions diminishes with increasing thermal kinetic energy 3kT/2, i.e., with increasing temperatures. The more accurate Van der Waals equation takes into consideration molecular size and attraction. The ideal gas law mathematically follows from statistical mechanics of primitive identical particles (point particles without internal structure) which do not interact, but exchange momentum (and hence kinetic energy) in elastic collisions.

1 Alternative forms
2 Proofs
- 2.1 Empirical
- 2.2 Theoretical
- 2.3 Derivation from the statistical mechanics
3 See also
4 References

[edit] Alternative forms

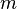Considering that the amount of substance could be given in mass instead of moles, sometimes an alternative form of the ideal gas law is useful. The number of moles ( $n\,$ ) is equal to the mass ( $\, m$ ) divided by the molar mass ( $\, M$ ):

$n = {\frac{m}{M}}$

Then, replacing $\, n$ gives:

$\ pV = \frac{m}{M}RT$

from where

$\ p = \rho \frac{R}{M}T$ .

This form of the ideal gas law is particularly useful because it links pressure, density $ρ = m / V$ , and temperature in a unique formula independent from the quantity of the considered gas.

In statistical mechanics the following molecular equation is derived from first principles:

$\ pV = NkT .$

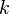Here $\,k$ is Boltzmann's constant, and $\,N$ is the actual number of molecules, in contrast to the other formulation, which uses $\,n$ , the number of moles. This relation implies that $N\,k = nR$ , and the consistency of this result with experiment is a good check on the principles of statistical mechanics.

From here we can notice that for an average particle mass of $μ$ times the atomic mass constant $m u$ (i.e., the mass is $μ$ u)

$N = \frac{m}{\mu m_\mathrm{u}}$

and since $ρ = m / V$ , we find that the ideal gas law can be re-written as:

$p = \frac{1}{V}\frac{m}{\mu m_\mathrm{u}} kT = \frac{k}{\mu m_\mathrm{u}} \rho T .$

[edit] Proofs

[edit] Empirical

The ideal gas law can be derived from Boyle's law, Charles's law, and Gay-Lussac's law.

Consider a gas sample with its initial state defined as:

volume =

V 0

pressure =

p 0

temperature =

T 0

If this gas undergoes an isobaric change, then from Charles's law the final state will be as follows:

volume: $V' = V_0(1 + \alpha T_0) \,$

pressure $p' = p_0 \,$

temperature $T' = T_0(1+\alpha T_0) \,$ .

If the gas then undergoes an isothermal change, Boyle's law gives

$pV = p_0V' \,$

where p is the final pressure and V is the final volume. Let T = T' designate the final temperature. Substituting in for V' produces

$pV = p_0V' = p_0V_0(1 + \alpha T_0) = {\frac{p_0 V_0}{T_0}}T$

The quantity ${\frac{p_0 V_0}{T_0}}$ is empirically found to be directly proportional to the amount of gas, measured in moles. The proportionality factor is designated $R$ and is called the universal gas constant.

Using this notation gives

$pV = nRT \,$

which is the ideal gas law.

[edit] Theoretical

The ideal gas law can also be derived from first principles using the kinetic theory of gases, in which several simplifying assumptions are made, chief among which are that the molecules, or atoms, of the gas are monatomic point masses, possessing mass but no significant volume, and undergo only elastic collisions with each other and the sides of the container in which both linear momentum and kinetic energy are conserved.

[edit] Derivation from the statistical mechanics

Let q = (q_x, q_y, q_z) and p = (p_x, p_y, p_z) denote the position vector and momentum vector of a particle of an ideal gas,respectively, and let F denote the net force on that particle, then

$\begin{align} \langle \mathbf{q} \cdot \mathbf{F} \rangle &= \Bigl\langle q_{x} \frac{dp_{x}}{dt} \Bigr\rangle + \Bigl\langle q_{y} \frac{dp_{y}}{dt} \Bigr\rangle + \Bigl\langle q_{z} \frac{dp_{z}}{dt} \Bigr\rangle\\ &=-\Bigl\langle q_{x} \frac{\partial H}{\partial q_x} \Bigr\rangle - \Bigl\langle q_{y} \frac{\partial H}{\partial q_y} \Bigr\rangle - \Bigl\langle q_{z} \frac{\partial H}{\partial q_z} \Bigr\rangle = -3k_{B} T, \end{align}$

where the first equality is Newton's second law, and the second line uses Hamilton's equations and the equipartition theorem. Summing over a system of N particles yields

$3Nk_{B} T = - \biggl\langle \sum_{k=1}^{N} \mathbf{q}_{k} \cdot \mathbf{F}_{k} \biggr\rangle.$

By Newton's third law and the ideal gas assumption, the net force on the system is the force applied by the walls of their container, and this force is given by the pressure P of the gas. Hence

$-\biggl\langle\sum_{k=1}^{N} \mathbf{q}_{k} \cdot \mathbf{F}_{k}\biggr\rangle = P \oint_{\mathrm{surface}} \mathbf{q} \cdot \mathbf{dS},$

where dS is the infinitesimal area element along the walls of the container. Since the divergence of the position vector q is

$\boldsymbol\nabla \cdot \mathbf{q} = \frac{\partial q_{x}}{\partial q_{x}} + \frac{\partial q_{y}}{\partial q_{y}} + \frac{\partial q_{z}}{\partial q_{z}} = 3,$

the divergence theorem implies that

$P \oint_{\mathrm{surface}} \mathbf{q} \cdot \mathbf{dS} = P \int_{\mathrm{volume}} \left( \boldsymbol\nabla \cdot \mathbf{q} \right) dV = 3PV,$

where dV is an infinitesimal volume within the container and V is the total volume of the container.

Putting these equalities together yields

$3Nk_{B} T = -\biggl\langle \sum_{k=1}^{N} \mathbf{q}_{k} \cdot \mathbf{F}_{k} \biggr\rangle = 3PV,$

which immediately implies the ideal gas law for N particles:

$PV = Nk_{B} T = nRT,\,$

where n=N/N_A is the number of moles of gas and R=N_Ak_B is the gas constant.